Paradoxes: The Box Problem: Remember the puzzle I posted a short time ago? I state it again…
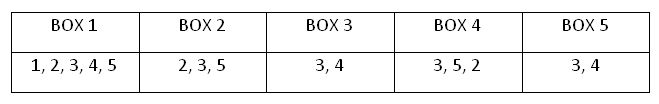
The boxes below contain the BOX numbers of five boxes:
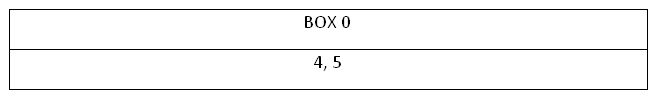
BOX 0 contains all BOX numbers that do not contain their own BOX numbers.
Here are two questions:
- Should 0 be in Box 0?
- Should 0 not be in Box 0?
The answers to the questions are as follows:
- If 0 is in Box 0, it should not be.
- If 0 is not in Box 0, it should be.
This is an example of paradoxes. Here are some others.
Paradoxes: The Barber Problem
The barber shaves all those who do not shave themselves. Who shaves the barber? Answer: if he shaves himself he should not; if he does not shave himself he should. Voila! A paradox.
Paradoxes: The Hanging Problem
This paradox is taken from Cervantes “Don Quixote”. A man is about to enter a town that only allows truth tellers to enter. There are guards at the entrance, and they ask a potential visitor to make a statement. If the statement is true he may enter; if it is false he is hung. Here is his statement: “You will hang me”. If the guards hang him, they should not; if they don’t hang him they should.
Paradoxes: The Party Problem
Consider the following statements:
A. Only one of these three statements is true
B. John will come to the party
C. John will not come to the party
All possible truth values of these statements are exhibited below:
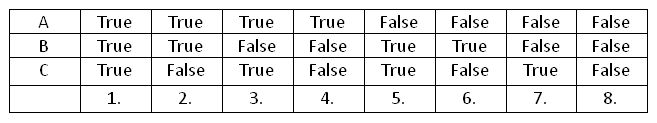Cases 1., 2., 3., 5., and 8. cannot hold because of A: “Only one of these three statements is true”.
Case 4. cannot hold because both B and C cannot both be false.
Cases 6. and 7. cannot hold since A is false so two or more of the statements must be true.
Do these three statements constitute a paradox? I have yet to come upon a mathematical definition that will allow me to decide. Suggestions?
Paradoxes: The Mysterious Doors Problem
You are stuck in a dungeon. You are dying of starvation. You look to your right, then look to your left. You see a door on each side of the room. To the left of you is an armored guard, and to the right of you is another armored guard.
One door leads to a mansion along side of a beach, where you can live a happy life. The other leads to a dumpster.
Once you go in one of the doorways, you can’t turn around and come. The guards and doors look identical. But one guard always lies, and the other always tells the truth. You don’t know which door leads to where, or which guard lies.
You can ask one question to just one of the guards. These guards are cruel to you, so they want you to end up in the dumpster. In order to get to the paradise, what do you ask the chosen guard? The answer is below written backwards.
ANALYSIS
Did you enjoy these paradoxes? You might also enjoy another paradox article written by a different mathematician, Mike DeHaan of Decoded Science. His piece is entitled
Zeno’s Paradox of Achilles and the Tortoise.
Note: You might also enjoy I’ve Got Your Number
← Back to Math-Logic-Design
← Home
